This website uses cookies to ensure a better user experience.
To get more information, please read our Cookie Statement.
Quantum vortex liquid in iron-based superconductors FeSe1-xSx and FeSe1-xTex with the electron nematic order
In a new paper published in Nature Communications, our colleague Matija Čulo in collaboration with scientists from England, Netherlands and Japan, reported a high-field magnetotransport study that provided compelling evidence for the existence of an exotic and a very rare quantum vortex liquid phase in superconductors FeSe1-xSx and FeSe1-xTex with the electron nematic order.
Expanded quantum vortex liquid regimes in the electron nematic superconductors FeSe1-xSx and FeSe1-xTex
M. Čulo, S. Licciardello, K. Ishida, K. Mukasa, J. Ayres, J. Buhot, Y.-T. Hsu, S. Imajo, M. W. Qiu, M. Saito, Y. Uezono, T. Otsuka, T. Watanabe, K. Kindo, T. Shibauchi, S. Kasahara, Y. Matsuda, N. E. Hussey.
Nature Communications 14, 4150 (2023). DOI: 10.1038/s41467-023-39730-9
Quantum vortex liquid is an exotic state of type-II superconductors, in which the standard Abrikosov vortex lattice is melted even at extremelly low temperatures (T), due to strong quantum fluctuations of the superconducting order parameter. Such a state is theoretically very poorly understood, and experimentally has been confirmed only in a few materials. One of the key questions is the exact origin of these strong superconducting quantum fluctuations and the role played by nearby non-superconducting phases.
In a new study, our colleague Matija Čulo in collaboration with scientists from England, Netherlands and Japan, provides compelling evidence for the existence of such a rare and exotic quantum vortex liquid state in iron-based superconductors FeSe1-xSx and FeSe1-xTex, which are unique due to unconventional superconductivity that emerges from a pure eletron nematic state. Presence of the quantum vortex liquid was indicated by determining two critical magnetic fields (H): the so-called melting field Hm, beyond which vortex lattice transforms into vortex liquid and upper critical field Hc2, beyond which the vortex liquid transforms into normal (non-superconducting) state. The critical fields Hm and Hc2 were extracted from the measurements of electrical resistance (R) in high magnetic fields up to 60 T and at very low temperatures down to 0.3 K in a way illustrated in Figure 1a) for FeSe1-xSx with x = 0.25 at T = 0.3 K.
The same procedure was carried out for all measured temperatures and for all FeSe1-xSx and FeSe1-xTex samples, and such determined Hm(T) and Hc2(T) were used for the construction of H-T phase diagrams in which the following phases can be discerned: vortex lattice/solid below Hm(T), vortex liquid between Hm(T) and Hc2(T) and normal (non-superconducting) state above Hc2(T). An example of such an H-T phase diagram is shown in Figure 1b) for FeSe1-xSx with x = 0.25. As we can see, there is a large separation between Hm(T) and Hc2(T) lines, which implies that the vortex lattice is melted and transformed into the vortex liquid across a significant part of the phase diagram, due to strong thermal fluctuations of the superconducting order parameter. Moreover, large separation between Hm(T) and Hc2(T) persists even at T → 0, where thermal fluctuations become negligible so that only quantum fluctuations can be responsible for destroying the vortex lattice. Such behavior provides compelling evidence for the existence of a quantum vortex liquid in FeSe1-xSx with x = 0.25.

Figure 1. a) Example of the determination of critical fields Hm and Hc2 from the measurements of electrical resistance R as a function of H for FeSe1-xSx with x = 0.25 at T = 0.3 K. For convenience, shown is the derivative of resistance dR/dμ0H normalized at 38 T, where μ0 is the vacuum permeability. Theoretically, Hm represents the field beyond which R ≠ 0, which in our case reduces to the field beyond which dR/dμ0H suddenly increases above 0. Hc2 is theoretically the field beyond which the normal (non-superconducting) state is established, which in our case reduces to the field beyond which the dR/dμ0H curve in the superconducting state (blue line at 0.3 K) starts to overlap with the dR/dμ0H curve in the normal state (red line at 10 K). b) Resulting H-T phase diagram for FeSe1-xSx with x = 0.25 with the following phases: normal (non-superconducting) state, vortex lattice/solid, vortex liquid and quantum vortex liquid.
Similar H-T phase diagrams were also obtained for the rest of FeSe1-xSx and FeSe1-xTex samples, indicating that the quantum vortex liquid regime is present for all S and Te compositions. How strong is the quantum vortex liquid can be determined from the ratio between the melting field and the upper critical field Hm(0)/Hc2(0), estimated in the limit T → 0 in the phase diagrams like the one in Figure 1b). The further the ratio Hm(0)/Hc2(0) from 1, the stronger the quantum vortex liquid regime. The dependence of such obtained ratio Hm(0)/Hc2(0) on x is shown in Figure 2 for both families FeSe1-xSx and FeSe1-xTex. As we can see, the quantum vortex liquid regime in FeSe1-xSx is the strongest outside of the nematic phase for x ≈ 0.25, and in FeSe1-xTex inside the nematic phase for x ≈ 0.30. Such behavior indicates that there is no simple correlation between superconducting quantum fluctuations, i.e. the quantum vortex liquid regime and the nearby (non-superconducting) nematic phase. On the other hand, Figure 2 clearly shows that there is a strong correlation between the quantum vortex liquid regime and the superconducting phase itself, since wherever the quantum vortex liquid is the strongest, the superconducting transition temperature Tc is the smallest. Here it should be stressed that this is not a trivial conclusion, since such an expanded quantum vortex liquid regime is never observed in conventional superconductors, which have small values of Tc. These results could therefore be the key for the understanding of this exotic and very rare state in unconventional superconductors.
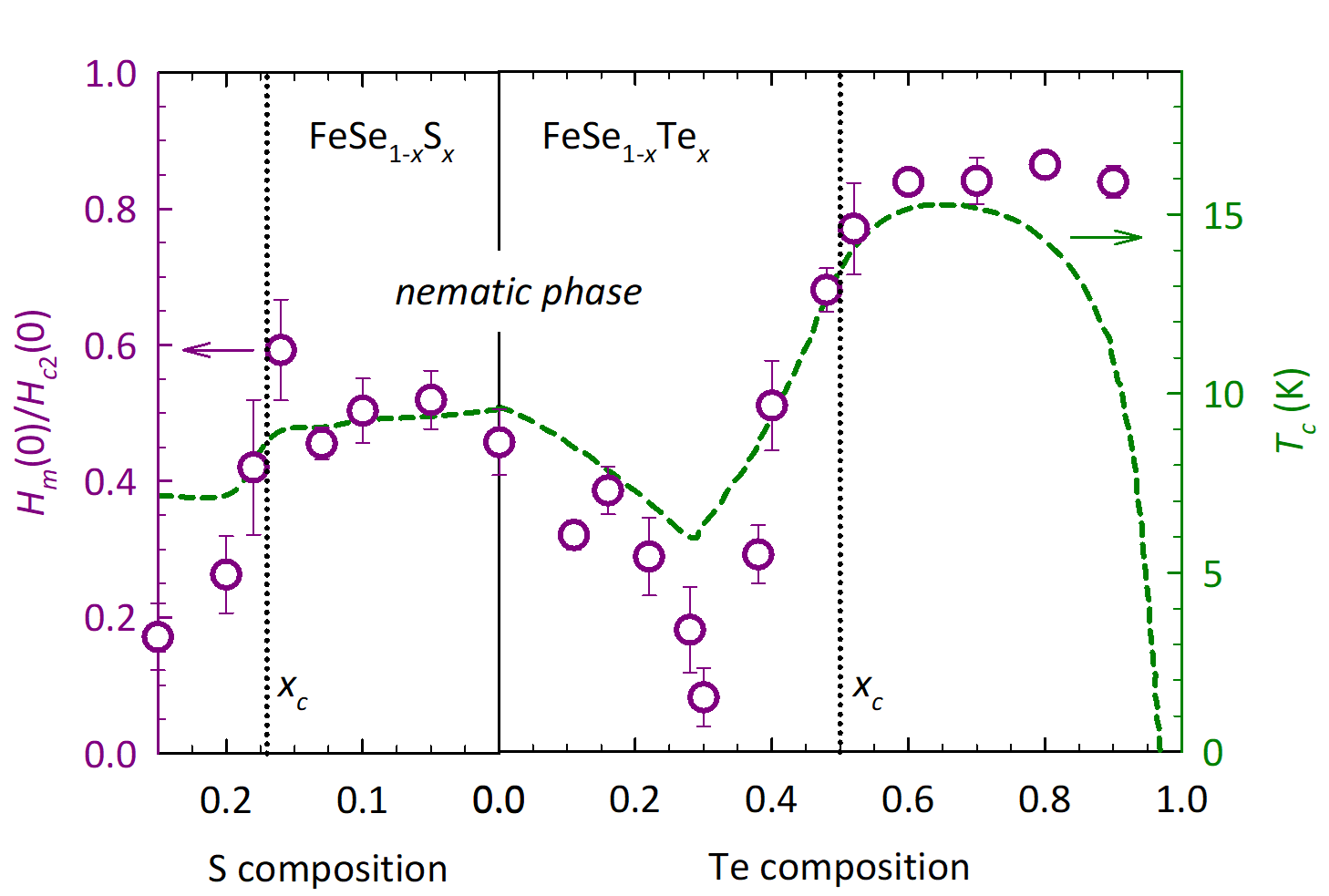
Figure 2. Evolution of the quantum vortex liquid regime with S and Te composition x in FeSe1-xSx and FeSe1-xTex. Vertical dotted lines marked xc represent the boundaries of the nematic phase. Violet symbols represent the strength of the quantum vortex liquid Hm(0)/Hc2(0) (left axis), and green dashed line represents the superconducting transition temperature Tc (right axis). The correlation between Hm(0)/Hc2(0) and Tc is obvious, implying that the quantum vortex liquid regime is the most pronounced wherever superconductivity is the weakest.